Trigonometry (I)
Mathematics SSS 1 Third Term
WEEK 5
Trigonometry (I)
Performance Objectives
Students should be able to;
- Identify ratios forming cosine and tangent of marked angle
- Draw right angle triangles
- Use right-angle triangles to solve problems involving calculations of lengths
Basic Trigonometric Ratios (Sine, Cosine, Tangent, with respect to right-angled triangles)
Content
These trigonometric ratios are applicable to the right-angled triangle. A right-angle triangle is 900. Thus the remaining two angles add up to 900 since every triangle contains two right angles.
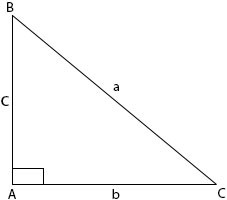
In ∆ABC, B + C = 900.
Such angles whose sum is 900 are said to be complementary angles. While capital letters are used for angles, small (lower case) letters are used for sides. Notice that the side opposite A is labelled a, the one opposite B is labelled b etc.
.... View MoreSubscribe now to gain full access to this lesson note
Take Me ThereClick here to gain access to the full notes.

